Page 35 - 《摩擦学学报》2020年第3期

第 3 期 侯硕, 等: 椭圆形织构摩擦片在核电站安全制动器中作用机理的数值研究 301

3
3
= p θ=0.5θ 1 ∂ ph ∂p ∂ ph ∂p U x ∂(ph) U y ∂(ph)
p θ =−0.5θ 1
( )+ ( ) = + (5)
∂x 12µ ∂x ∂y 12µ ∂y 2 ∂x 2 ∂y
where (x, y) is the coordinate in the rectangular
r o
coordinate system, U , U is the velocity along the x and
x
y
β
o θ 1 y directions respectively, p is pressure of gas film. μ is
r i
viscosity of gas film, h is thickness of gas film.
p i
p o
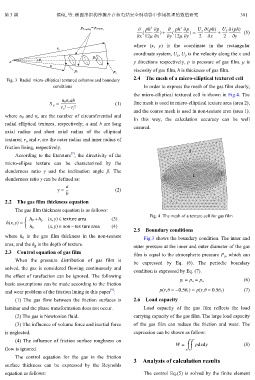
2.4 The mesh of a micro-elliptical textured cell
Fig. 3 Radial micro-elliptical textured columns and boundary
conditions In order to express the mesh of the gas film clearly,

the micro-elliptical textured cell is shown in Fig.4. The
n θ n r ab
S p = (1) fine mesh is used in micro-elliptical texture area (area 2),
2 2
r o −r i
and the coarse mesh is used in non-texture area (area 1).
where n and n are the number of circumferential and
θ
r
In this way, the calculation accuracy can be well
radial elliptical textures, respectively; a and b are long
ensured.
axial radius and short axial radius of the elliptical

textures; r and r are the outer radius and inner radius of
o i
friction lining, respectively.
1
[5]
According to the literature , the directivity of the
micro-ellipse texture can be characterized by the
slenderness ratio γ and the inclination angle β. The
2
slenderness ratio γ can be defined as:
a
γ = (2)
b
2.2 The gas film thickness equation
The gas film thickness equation is as follows:
Fig. 4 The mesh of a texture cell for gas film
{
h 0 +h p (x,y) ∈ texture area (3)
h(x,y) =
h 0 (x,y) ∈ non−tex ture area (4)
2.5 Boundary conditions
where h is the gas film thickness in the non-texture Fig.3 shows the boundary condition. The inner and
0
area, and the h is the depth of texture. outer pressure at the inner and outer diameter of the gas
p
2.3 Control equation of gas film
film is equal to the atmospheric pressure P , which can
a
When the pressure distribution of gas film is
be expressed by Eq. (6). The periodic boundary
solved, the gas is considered flowing continuously and
condition is expressed by Eq. (7).
the effect of rarefaction can be ignored. The following
p i = p o = p a (6)
basic assumptions can be made according to the friction
[9] p(r,θ = −0.5θ 1 ) = p(r,θ = 0.5θ 1 ) (7)
and wear problem of the friction lining in this paper :
(1) The gas flow between the friction surfaces is 2.6 Load capacity
laminar and the phase transformation does not occur. Load capacity of the gas film reflects the load
(2) The gas is Newtonian fluid. carrying capacity of the gas film. The large load capacity
(3) The influence of volume force and inertial force of the gas film can reduce the friction and wear. The
is neglected. expression can be shown as follow:
(4) The influence of friction surface roughness on "
W = pdxdy (8)
flow is ignored.
The control equation for the gas in the friction
3 Analysis of calculation results
surface thickness can be expressed by the Reynolds
equation as follows: The control Eq.(5) is solved by the finite element